表面自由能、液体极性和固体胶粘剂中表征方法检测方案(接触角测量仪)

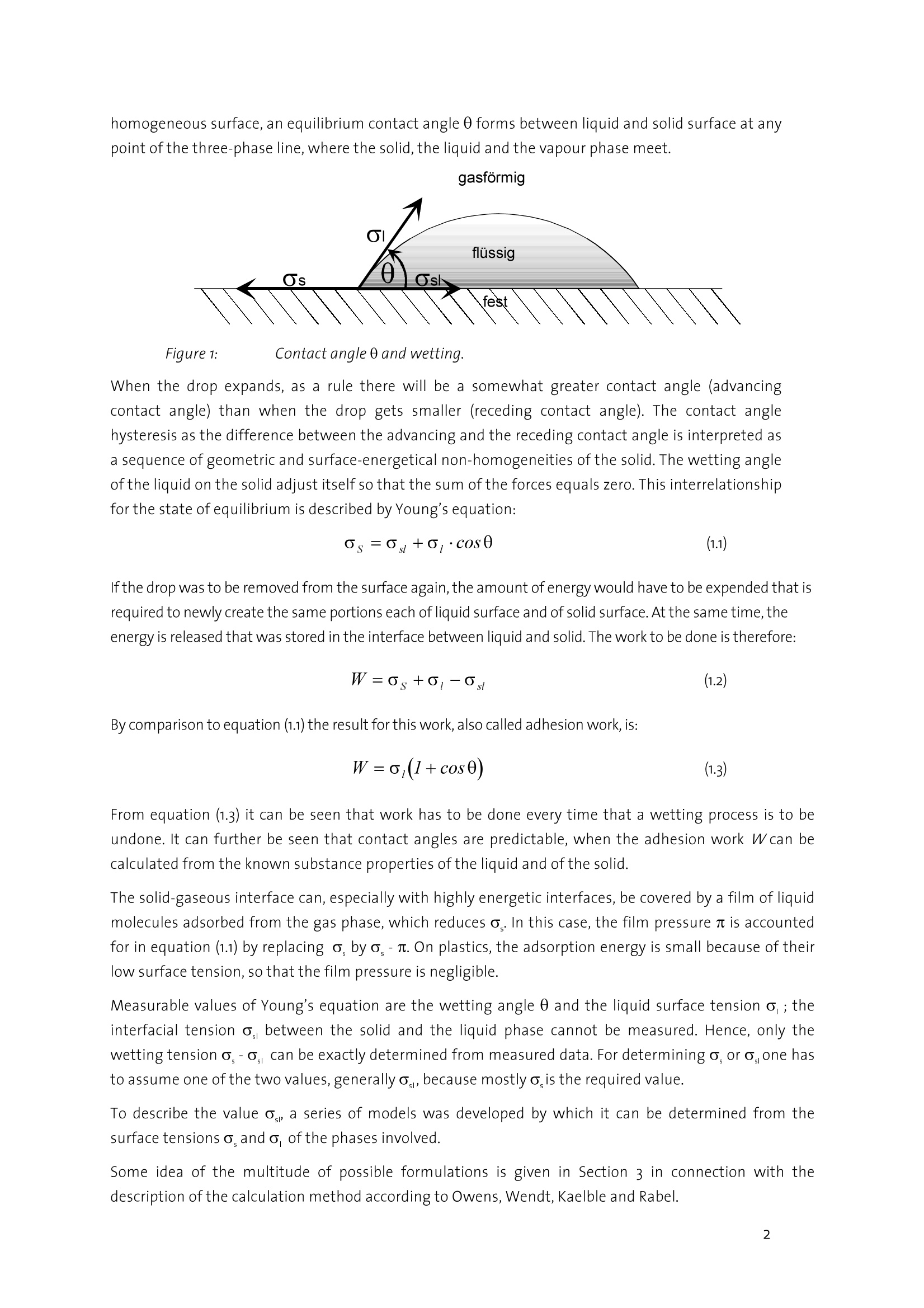
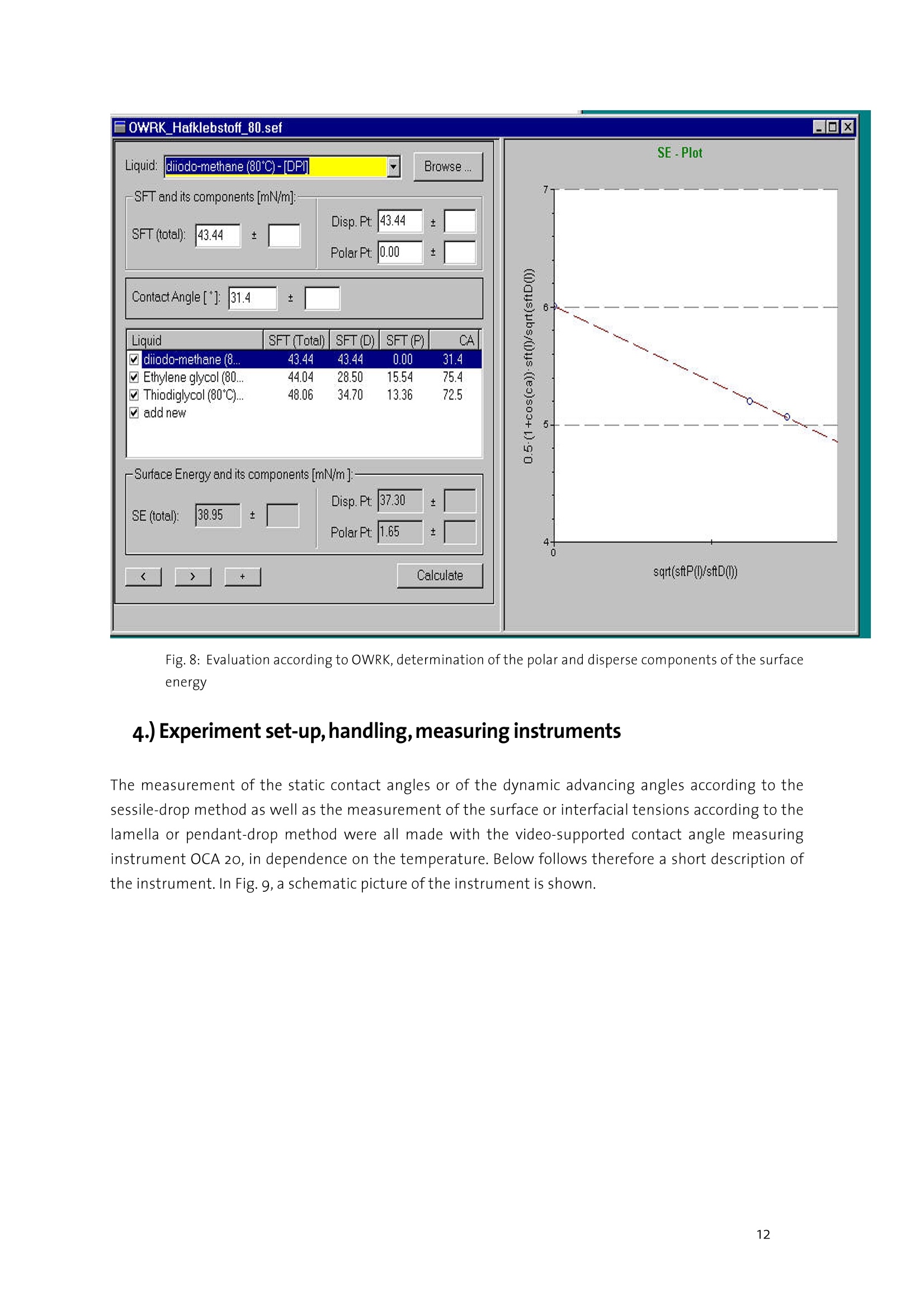
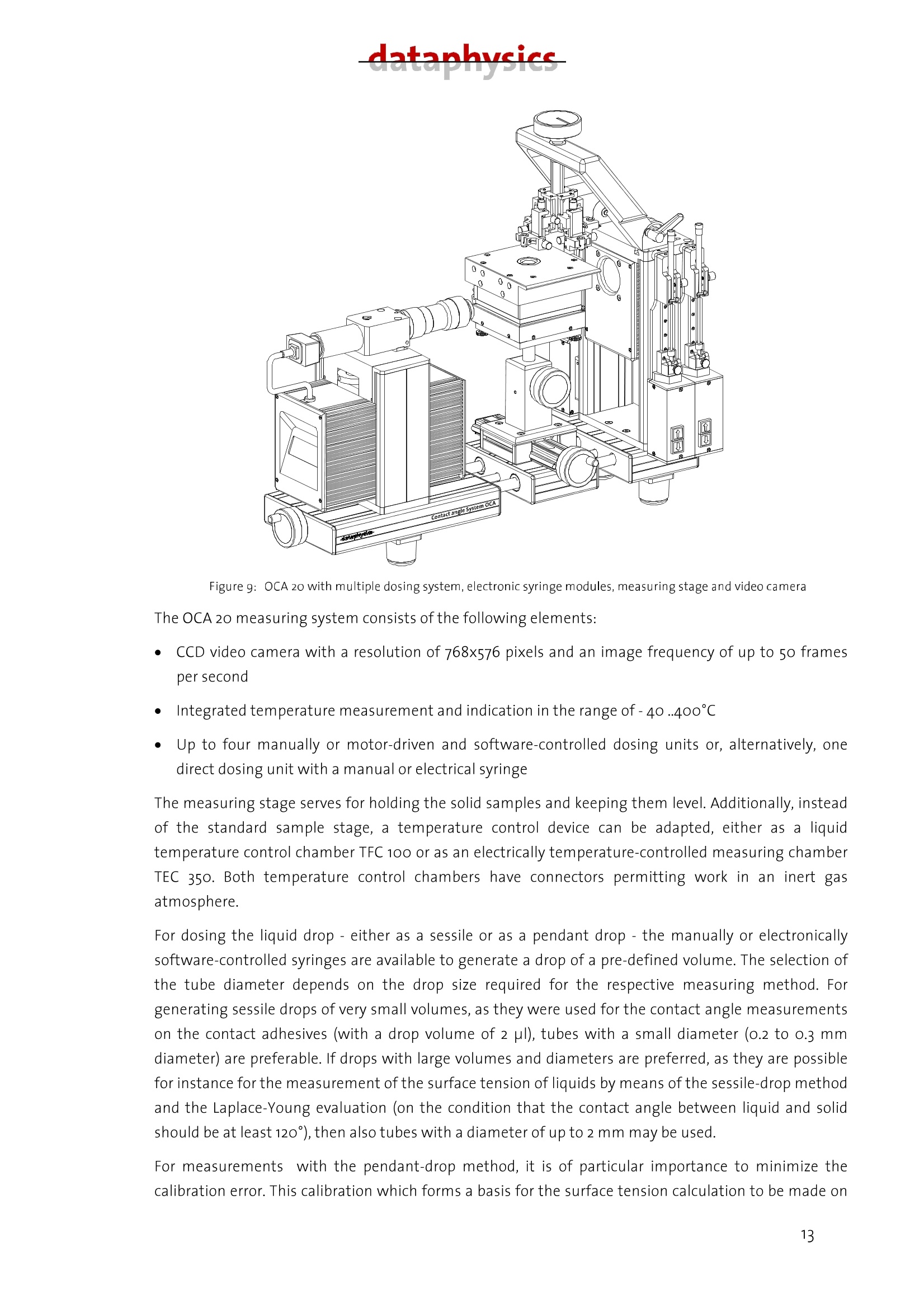
datanhucics Description of the analytical method forcharacterizing the surface energy and polarity ofliquid and solid contact adhesives 1. Basics Contact adhesives represent a complicated mixture of polymeric components， by which adhesion，cohesion， aging resistance and other properties can be controlled within wide limits. For theprocess of glueing， the knowledge of the surface energy of both adhering components and theirpolarities is of particular importance to avoid mis-matchings when selecting an adhesive. As anintroduction to the problems involved， the interface-physical basics of wetting are representedhere as one of the essential conditions for adhesion. Interfacial tension The interfacial tension can be introduced by means of thermodynamics. lf a condensed phase formsan interface with another phase or the vacuum， then the physical properties of the interface mustbe in incorporated in the description of the energetic behaviour of the phase. The value y(or o) is called the interfacial (or surface) tension and equals the reversible work thatmust be done to increase the interface. Its dimension is N/m， which is formally equivalent to theinterfacial energy (J/mo). Both terms are often equated， which， strictly spoken， is only true underisothermal conditions and is permitted for solid bodies when the mechanical energy for structuralchanges is negligible. In the following chapters， with an interface between two condensed phases， o is called theinterfacial tension， with an interface between a condensed and a gas phase or the vacuum it iscalled surface tension. The interfacial tensions are a direct consequence of the intermolecular forces of interaction. The surface or interfacial tensions of liquids can be directly measured with the known tensiometricmethods or the pendant-drop method described below. Surface tensions of solids， however， can only be determined indirectly. The most frequently appliedmethod to determine the surface tension of solids with low surface energy (such as plastics) is thedetermination from the wetting angle data. Contact angle The wetting angle (also known as contact angle) between a drop of a liquid with a known surfacetension and a solid surface (see Fig. 1) depends on the relation between the adhesive forces， whichwould make the drop spread on the surface， and the cohesive forces of the liquid， which wouldcontract the drop to a sphere with a minimum surface. lf a drop of liquid lies on an ideally level， homogeneous surface， an equilibrium contact angle 0 forms between liquid and solid surface at anypoint of the three-phase line， where the solid， the liquid and the vapour phase meet. Figure 1： Contact angle 0 and wetting. When the drop expands， as a rule there will be a somewhat greater contact angle (advancingcontact angle) than when the drop gets smaller (receding contact angle). The contact anglehysteresis as the difference between the advancing and the receding contact angle is interpreted asa sequence of geometric and surface-energetical non-homogeneities of the solid. The wetting angleof the liquid on the solid adjust itself so that the sum of the forces equals zero. This interrelationshipfor the state of equilibrium is described by Young's equation： lfthe drop was to be removed from the surface again，the amount of energy would have to be expended that isrequired to newly create the same portions each of liquid surface and of solid surface. At the same time，theenergy is released that was stored in the interface between liquid and solid. The work to be done is therefore： By comparison to equation (1.1) the result for this work， also called adhesion work， is： From equation (1.3) it can be seen that work has to be done every time that a wetting process is to beundone. It can further be seen that contact angles are predictable， when the adhesion work W can becalculated from the known substance properties of the liquid and of the solid. The solid-gaseous interface can， especially with highly energetic interfaces， be covered by a film of liquidmolecules adsorbed from the gas phase，which reduces o. In this case， the film pressure n is accountedfor in equation (1.1) by replacing o， by o，-. On plastics， the adsorption energy is small because of theirlow surface tension， so that the film pressure is negligible. Measurable values of Young's equation are the wetting angle 0 and the liquid surface tension o； theinterfacial tension o， between the solid and the liquid phase cannot be measured. Hence， only thewetting tension o，-o can be exactly determined from measured data. For determining o， oro， one hasto assume one of the two values， generally o，， because mostly o，is the required value. To describe the value o，， a series of models was developed by which it can be determined from thesurface tensions o and o of the phases involved. Some idea of the multitude of possible formulations is given in Section 3 in connection with thedescription of the calculation method according to Owens， Wendt， Kaelble and Rabel. datanhucics. 2.) Measuring method to determine contact angles， surface and interfacialtensions To calculate the surface energy of a contact adhesive， in case of a change of phase within thetemperature range to be measured， several measuring methods are necessary. In the temperature rangewhere the contact adhesive is in a solid state， the calculation of the surface energies is made on the basisof contact angle measurements with 3 liquids of different polarities on adhesive layers prepared fromthe molten mass. In the upper temperature range， where the contact adhesive is in a liquid state， for the calculation of thedisperse and the polar portions of the surface energies， measurements of the surface tensions directlyon the molten contact adhesive are made with the lamella method and measurements of the interfacialtension of the molten contact adhesive against a purely disperse solvent with the pendant-drop method. Measurement of contact angles with the sessile-drop method In the past and still today， contact angles are mostly determined by means of optical methods in atransmitted light set-up. For this purpose， a resting drop is exposed to diffused light from one side andobserved from the other side. In the most simple set-up this measurement is made by means of aprotractor eyepiece， in which case the result may be influenced by the subjective perception of theexperimenter and the results are therefore hardly reproducible. This is why recently， video-supportedsystems are increasingly used. This is enabled not least by the availability of high-performance imageprocessing cards and reasonably priced powerful computers. In these systems， the eyepiece is mostly replaced by a CCD camera. In order to determine the contactangle， the image processing software has to perform the following tasks， which are schematically shownin Fig.2： Figure2： Schematic illustration of the measuring process of contact angles ●Cdetermination of the base line at the phase boundary solid/liquid determination of the drop contour measurement of the contact angle First， by means of the CCD camera a digital image of the drop on the surface is recorded.The position of the base line and also that of the drop contour is determined bycalculating the difference of the brightness of one image spot to the adjacent imagespot. The drop contour and the base line then result from the position of the maximumdifferences between brightnesses，i.e. of the maximum contrast. ●Before determining a contact angle， the drop contour line must be matched to themeasured drop outline. Various methods are applied for this，which differ considerably intheir accuracy， but also in the amount of calculation they require. In the height/width method， a segment of a circle is matched to the drop outline.Especially with larger drops， this method leads to considerable errors， because due togravity， the drop shape greatly differs from that of a spherical segment. .In the ellipse method， a line of elliptical shape is matched to the drop outline. Here， thedeviations from the actual shape of the drop are a few percent. In the Young-Laplace method，a curve is matched that exactly follows the drop outline.The drop shape is determined by the force equilibrium between surface tension andgravity. In the Young-Laplace method， the corresponding equation is solved numerically，with the solution being adapted to the previously determined drop outline by means of aparameter. A comparison of the various methods shows that only the ellipse and the Young-Laplacemethod yield satisfying accuracies in determining the contact angle. The ellipse methodhas the advantage of supplying a result within fractions of a second， whereas the Young-Laplace method， depending on the computer used， requires 1-2 seconds. For rapidabsorption processes， this method is therefore only useful up to a point. To determine the surface energy of a solid with its polar and disperse portions， the contact angle ismeasured with a number of test liquids and evaluated with a suitable， pre-defined method. In thevideo-supported contact angle measuring instrument OCA 20 of Dataphysics， which is to bedescribed below， the evaluation method according to Fowkes， Owens-Wendt， Wu， Neumann， theextended Fowkes method and the option to evaluate after the Bronstedt-Lowry theory areintegrated in the software and are (after selection) performed fully automatically by the computer.For the case of pure polymer materials， the method according to Wu has proven useful， because -as known from literature - especially with low-energetical systems (as represented by polymers) itleads to better reproducible results than other methods. For the case of multi-component plasticsystems， the determination of the solid surface tension after the Owens-Wendt method has datanhucics proven useful. The method according to Wu requires the use of at least two， the method accordingto Owens-Wendt of at least two， better three suitable test liquids， and each additional liquidincreases the accuracy of the calculated result. The choice of the test liquids has a crucial influenceon the accuracy of the values obtained， the selection of suitable test liquids， however， cansometimes be difficult. On the one hand， an influence of the test liquid on the surface by partiaetching or dissolving must be excluded. On the other hand， the surface tension of the test liquidmust not be altered by possibly existing soluble substances on the surface. If one or both of thedescribed processes are found， this shows in a wide variation of the measured contact angles inspite of a visually homogeneous surface， and in a bad reproducibility of the results. As a rule， wateris also used as a test liquid. This has to be ruled out， however， if for instance in the treatment ofplastics， water-soluble groups form on the surface， on which water molecules settle down andwhich therefore falsify the measurement. Alternatively，innsuch cases：sethylene glycol，diiodomethane， dimethyl sulphoxide (DMSO) and formamide are used. In the case of contact angle measurements on the contact adhesives in their solid state，the selectionof solvents has been specified in so far as diiodomethane or p-cymene are used as disperse liquids.Decisive for the selection of these particular solvents as reference liquids， beside chemicalconsiderations， were their thermal stability as well as their pure dispersivity (with diiodomethane)and their varying polar portions (for ethylene glycol and thiodiglycol) with total surface tensions of asimilar order of magnitude. Hence， the demand for great polarity differences of the test liquids as oneof the pre-conditions for determining solid surface energies from wetting angle measurements isfulfilled. After having optimized these marginal conditions， the experimental procedure of contact anglemeasurement is reduced to an exact dosing of the liquid (by means of a manual or automatic dosingsystem) and the generation of a drop suited for evaluation as well as the exact optical recording (in apossibly optimal bright-dark contrast if recorded as a digital image by means of a CCD camera) and theevaluation of the drop contour by means of the software. An example for an evaluable contour of asessile drop is given in Fig.3. Figure 3： Contour of a sessile drop The pendant-drop method The method of the pendant drop is based on the generation of a well-formed drop and the opticalrecording and evaluation of its contour. lf the drop is in contact with the surrounding gas space， the surface tension of the liquid generating thedrop can be determined. If the adjacent phase is formed by a second liquid， the interfacial tensionexisting between the two liquids can be found by determining the the drop profile. The knowledge ofthe surface tension of the liquid phase in relation to the surface tension of the solid is - as alreadyconsidered in the introduction - a necessary criterion for making predictions regarding wettingprocesses. A very crucial criterion (especially for adhesion， too) are the polar and the disperse portions ofthe surface or interfacial tension of the liquid (or of the non-setting contact adhesives， which areregarded as high-viscosity liquids also at room temperature， where a sufficiently great molecularmobility can be assumed). if the adhesive and the surface to be glued have the same or very similaroverall surface tensions， good glueing properties may be expected only if the polarity is nearly thesame. The knowledge of the polarity becomes less important when the overall surface tensions differconsiderably. Hot-setting contact adhesives were taken as an example to find， on the basis of pendant-dropmeasurements， the interfacial tension between the hot-setting contact adhesives and a purely dispersesolvent and to determine the polar and disperse portions of the surface tension of the contactadhesives by means of a calculation method to be explained below (see Section3). For this purpose， adrop of the liquid to be investigated is generated at the bottom end of a capillary with a manual orautomatic dosing system. In the case of a liquid as the surrounding medium， the liquid with the higherdensity should be placed in a cuvet， and the liquid with the lower density should form the drop withinthis phase. With a CCD camera，the contour of the drop (Fig.4) is recorded. Figure 4： Contour of a pendant drop. The outer shape of the drop is determined by two forces. For one thing， the weight acts on the length ofthe drop in vertical direction， for the other， the surface tension strives to keep the drop in spherical shapeto minimize its surface. Characteristic of the state of equilibrium is the change of the curvature along thedrop contour. This equilibrium of forces is described in a mathematically exact way by the Young-Laplaceequation. If the contour of the drop is known， the surface or interfacial tension can be determined bysolving this equation. The disperse or the non-polar portion of the surface tension can be calculated by datanhucics measuring the interfacial tension of the test liquid against a completely non-polar liquid. The polarportion then results from the difference between the overall surface tension and the disperse portion. For hot-setting contact adhesives it is shown how by means of the contact angle measuring instrumentOCA20 the polar and disperse portions of their surface tension can be easily determined on the basis ofthe pendant-drop method. As a purely disperse liquid for the required interfacial tensions， FC7o waschosen. The presented method is of fundamental interest and can be applied to any liquid whosewetting properties are to be characterized. The surface tension o of the contact adhesive is determined with the OCA20 and the SCA22 software inair. To calculate the non-polar portion， the interfacial tension o，， is measured against FC 70(perfluortripentylamine). The FC 70 as a purely disperse liquid was chosen because the contactadhesives are practically insoluble in it， whereas by many other disperse liquids， depending on thetemperature they are more or less strongly etched or completely dissolved. According to Owens & Wendt， the following applies for the interfacial tension o， ： Where o，" and o， mean the disperse and the polar portion of the investigated PSAs， whereas o，" and o，represent the respective portions of the non-polar surrounding medium， in this case FC 70. Hence o，"=o，， because o，"=o. Therefore the disperse portion is determined by transposing the equation (1.4)： The polar portion is calculated according to： A problem may occur if the interfacial tension of the contact adhesive against the purely disperse liquidis lower than the surface tension of the disperse liquid. In this case the calculation of the disperse andthe polar portions ofthe surface energy of the contact adhesive using the Owens-Wendt equation to beexplained below becomes problematic. It would then have to be checked whether a different method -like for instance the evaluation according to Wu-should be applied. Summing up， it can be said that with the contact angle measuring instrument OCA 2o and thepertaining software SCA 22 on the basis of the pendant-drop method there exists a very precise andrapid way to characterize a liquid in general and contact adhesives in particular regarding their wettingproperties. The surface tension is directly accessible， and the polar and disperse portions can becalculated relatively easily after having determined the interfacial tension against a non-polar liquid. From the knowledge of the polar and disperse portions of the surface tension of the contact adhesivesunknown so far， predictions regarding wetting and adhesion processes are possible. Method with the DataPhysics OCA 20 Lamella method The lamella method developed by DataPhysics represents a new method of determining the surfacetension at increased temperatures. The determination of the surface tension of molten polymers orcontact adhesives so far was only possible with considerable experimental efforts. The lamellamethod permits a rapid and precise measurement of the surface tension with a considerably reducedexperimental effort compared to conventional methods， even at temperatures up to 350°C. Traditional methods of determining the surface tension with gravimetric tensiometers are mostlyrestricted to a temperature range up to max.100℃. In suitable temperature control devices， thecontour analysis of pendant drops (pendant-drop method) is also possible at higher temperatures.What is problematic is the handling of high-viscosity molten polymers. The dosing by means ofheated syringes is troublesome， and especially their cleaning is difficult and time-intensive. Moreover，problems frequently occur from the formation of gas bubbles in the syringe， which make the dosingof drops of a defined size difficult or even impossible. The newly developed lamella method avoidsthese difficulties， because only easy to clean components come into contact with the molten polymer，and the samplecontainer is open so that gas inclusions cannot occur. Like the method of the pendant drop， the lamella method is based on the equilibrium between theweight and the surface tension. As schematically shown in Fig. 5， a liquid lamella will form if a verticaltest body is brought into contact with a liquid. With contact angles between o° and 90°， the lamella iscurved upward， whereas for contact angles between 9o°and 18o° it is curved downward. Figure5. Schematic representation of the formation of a lamella If the contact angle is exactly9o°，then there is no curvature of the liquid. The lamella develops from the tendency of the liquid to wet the test body. In this process， the effect ofthe surface tension strives to keep the newly generated surface as small as possible. At the same time，the weight wants to minimize the volume of a rising lamella. At equilibrium， the effect of the surfacetension and that of the weight just counterbalance each other. When the weight is known， the surfacetension of the liquid can be calculated from the exact knowledge of the lamella contour. The underlyingequation of the state of equilibrium is the differential Young-Laplace equation， for which there is noclosed analytical solution with these marginal conditions. By means of quick PC systems andaccordingly optimized solution algorithms， however， it is possible to work out the surface tensionexactly numerically within fractions of a second. From Fig.5 it becomes clear that with a contact angleof 90°and a straight cylindrical test body， no lamella can be generated. If，however， instead of a datanhucics cylindrical body a sphere is used， then a lamella will also form at a contact angle of 9o°， because thecurved spherical surface does not assume a constant go° angle towards the horizontal. The experimental procedure for the lamella method can be classified as follows. In a specially shapedsample container， the polymer or the contact adhesive is molten. For this purpose， the electricallyheated temperature control device TEC 350 is used， which in this case replaces the sample stage of theoptical contact angle measuring instrument OCA 20. Into the molten sample， the spherical test body isimmersed， so that its end is completely wetted. With the CCD camera a digital image of the lamella atthe required temperature is recorded and saved. To calculate the surface tension， the software mustwork out the weight of the lamella. For this it is necessary to enter the density of the molten materialand to determine the enlargement ratio of the recorded image. The density is entered by theexperimenter， whereas the enlargement ratio is worked out by means of the geometry of the test body.After the measurement， the test body as well as the sample container can be easily cleanedmechanically， for instance in an ultrasonic bath. By the example of a viscous contact adhesive， below the lamella method is compared to that of thependant drop. For this purpose， Fig. 6 shows the image of a pendant drop of the contact adhesive at120℃. Because of its rectangular format， the CCD camera was moved by 9o°from the horizontal toachieve the best possible image filling. Fig. 6： Pendant drop of a contact adhesive at 120℃. From this image， a surface tension of 35，16±0，04 mN/m was determined. A density of 1，0518 g/cmwasassumed. The lamella method was applied to the same material. The corresponding image is shown in Fig. 7. Thelamella and the spherical test body are clearly visible. Fig.7： Lamella of the contact adhesive at 120℃ From this image， a surface tension of 34，81± 0，35 mN/m for the same density as in Fig. 6 wasdetermined. Hence the two values agree within an error of 1% of the lamella method. 3.) Evaluation of the disperse and polar portions of the surfaceenergy At an interface， a particle experiences attractions from its own and from the adjacent phase. Amechanical consideration therefore supplies， for the interfacial tension， the difference between thesurface energies of both phases.This is expressed in the interfacial tension model of Antonow.On non-polar substances， the predictions from the Antonow model agree well with the experimental data. Asimilar train of thought forms the basis of Zisman's surface critical energy o： a liquid will completelywet asolid， when the forces of attraction of the solid phase acting on the liquid molecules are greaterthan those of the liquid phase. A solid has precisely the surface tension of the measuring liquid， whenits surface tension is just small enough to wet the solid completely. Fowkes suggested to divide the interfacial tensions between two condensed phases into additivecomponents orienting themselves on the individual intermolecular forces of interaction. He separatedthe disperse portion o containing London’s dispersion interactions， from the polar portion o Pcontaining the Keesom (dipole-dipole)， the Debye (dipole-induced dipole) and the hydrogen bridgeforces of interaction： From the Good-Girifalco equation， Owens and Wendt， considering the division of the interfacial tensionto equation (3.1)， developed a method with which the disperse and the polar surface tension portion ofsolid surfaces can be determined from wetting angle measurements with measuring liquids ofdifferent polarities. Wu replaced the geometric mean in the equations by the harmonic mean， which heexplained. Apart from this， his method corresponds to that of Owens and Wendt， which is to beconsidered more thoroughly here， because it forms the basis for the calculation of the surface energiesof the solid contact adhesives. The method according to Owens， Wendt，Rabel and Kaelble lOwens， Wendt， Rabel and Kaelble based their method on the assumption that the interfacial energycan be split up between the molecules according to the forces of interaction. They distinguish betweendisperse interactions and polar interactions. Polar interactions include the Coulomb interactionsbetween permanent dipoles and those between permanent and induced dipoles. The interaction due tofluctuations over time in the load distribution within the molecules is called disperse interaction. Polarand disperse contribution to the surface energy or surface tension are combined by addition. Hence itapplies： datanhucicsS- Where oand o" are the disperse and polar portions of the liquid， whereas o， and o， mean the respectiveportions of the solid. According to Owens， Wendt， Rabel and Kaelble， the interfacial energy can be calculatedfrom the portions of the liquid and of the solid by forming the geometric mean. It results foro If this expression is entered in equation (3.2) for o， and transposed towards the unknown values， the result isa straight line equation of the form where Hence， by protrating y against x， o can be calculated from the gradient of a compensation straight lineand o from the axial section. For this purpose， the contact angle of at least two liquid drops ismeasured on the unknown surface. If suitable computer software is available， the solution is calculated after entering the measuredcontact angles and the surface tension data of the liquids used within fractions of a second. By usingsuitable data bases with stored data on liquids over the temperature， the process is further simplified. Atypical evaluation using a computer is shown in Fig. 8. Fig.8： Evaluation according to OWRK， determination of the polar and disperse components of the surfaceenergy 4.)Experiment set-up，handling，measuring instruments The measurement of the static contact angles or of the dynamic advancing angles according to thesessile-drop method as well as the measurement of the surface or interfacial tensions according to thelamella or pendant-drop method were all made with the video-supported contact angle measuringinstrument OCA 20， in dependence on the temperature. Below follows therefore a short description ofthe instrument. In Fig.9， a schematic picture of the instrument is shown. datanhucics Figure g： OCA 20 with multiple dosing system， electronic syringe modules， measuring stage and video cameraThe OCA 20 measuring system consists of the following elements： CCD video camera with a resolution of 768x576 pixels and an image frequency of up to 5o framesper second Integrated temperature measurement and indication in the range of-40 ..400℃ Up to four manually or motor-driven and software-controlled dosing units or， alternatively， onedirect dosing unit with a manual or electrical syringe The measuring stage serves for holding the solid samples and keeping them level. Additionally， insteadof the standard sample stage， a temperature control device can be adapted， either as a liquidtemperature control chamber TFC 10o or as an electrically temperature-controlled measuring chamberTEC 350. Both temperature control chambers have connectors permitting work in an inert gasatmosphere. For dosing the liquid drop- either as a sessile or as a pendant drop - the manually or electronicallysoftware-controlled syringes are available to generate a drop of a pre-defined volume. The selection ofthe tube diameter depends on the drop size required for the respective measuring method. Forgenerating sessile drops of very small volumes， as they were used for the contact angle measurementson the contact adhesives (with a drop volume of2 pl)， tubes with a small diameter (0.2 to o.3 mmdiameter) are preferable. If drops with large volumes and diameters are preferred， as they are possiblefor instance for the measurement of the surface tension of liquids by means of the sessile-drop methodand the Laplace-Young evaluation (on the condition that the contact angle between liquid and solidshould be at least 120°)， then also tubes with a diameter of up to 2 mm may be used. For measurementswith the pendant-drop method， it is of particular importance to minimize thecalibration error. This calibration which forms a basis for the surface tension calculation to be made on the drop profile， is performed by the software integrated in the OCA 20 by means of the outer tubediameter independently determined prior to measuring (and which was previously entered in theevaluation software as a reference value). For liquids with surface tensions up to 30 mN/m， tubes witha diameter in the range from 1 to 2 mm， for liquid surface tensions in the order of magnitude of thewater value， tube diameters in the range from 1.5 to 3mm should be used. The recording and evaluationof the drop contour are automatically performed by the SCA 22 software. As another required value to be entered in advance， the density of the liquid with which the wettingangle measurements are made， as well as its surface tension with the polar and disperse portions at therespective measuring temperature must be known. If interfacial tension measurements are made withthe pendant-drop method， the knowledge of the densities of both liquids and their surface tensions atthe respective measuring temperature is required. For selected liquids， this information is included inthe integrated software of the OCA 20 in the form of a data base， which can be expanded at any time. Finally here are some remarks on handling the hot-setting contact adhesives. The measurement of the contact angles on the contact adhesives in their solid state according tothe sessile-drop method was directly made on the adhesive layers prepared from the molten massby determining the dynamic advancing angle. For this purpose， a piece of contact adhesive wasremoved from the respective compact material mechanically and under heat and then by meansof a sliding process directly molten onto a glass slide as evenly as possible. It is important to makethis molten layer as even as possible and without the inclusion of air. The glass slide with themolten layer to be measured was fixed in the liquid temperature control chamber TFC 100 or， from50℃ on， in the electrically temperature-controlled chamber TEC 350 and the temperature in thegas space surrounding the drop was determined. It has proven indispensable to work under drynitrogen in the temperature control chamber， because the contact adhesive layers molten ontothe glass slide tend to attract water very quickly. As liquids of different polarities， ehtylene glycol， thiodiglycol and diiodomethane were used.Diiodomethane as a purely disperse solvent turned out to not be suited for all kinds of hot-settingadhesives due to the etching effect visible on some contact adhesives. For certain contactadhesives， therefore， the contact angle measurements were additionally made with p-cymene as adisperse solvent and the resulting contact angle data incorporated in the calculation of the surfaceenergies. The samples， however， also show etching effects with the polar liquids ethylene glycoland， even more， with thiodiglycol. Some contact adhesives obviously containing tensides showedthese etching effects even at low temperatures. Due to the lacking constancy of the contact anglevalues， this made the determination of the surface energy more difficult. Therefore， in the courseof the measuring procedure it was decided to determine static contact angles in the phase of thefirst wetting of the molten layer. A practical replacement by other polar solvents not etching thecontact adhesives with a surface tension in a similar order of magnitude and the correspondingtemperature stability seems impossible at present. The evaluation of the surface energies of the contact adhesives in their liquid state by directmeasurement of their surface tensions was made on the OCA 20 with the lamella method by opticallymeasuring the curve contour of the lamella generated from the freshly molten contact adhesive (three-phase contact line) over the temperature. datanhucicsS- The respective contact adhesive sample was mechanically removed from its compact material， molten ina specially made metal cuvet and then fixed in the electrically temperature-controlled chamber TEC 350.The temperature in the gas space surrounding the molten mass was determined. It is recommended towork under dry nitrogen. By means of a rotationally symmetrical sample body (whose diameter must bedetermined prior to the measurement by means of a micrometer screw)， a lamella is generated from thismolten mass above the contact adhesive reservoir and - as described- optically measured and evaluated.The results of the automatic contour evaluation were average values of the measured surface tensionsfor the respective hot-setting adhesive over the temperature. For the lamella method， in addition to the diameter of the test body (on which the molten lamella isdrawn from the reservoir)， also the density of the molten mass at the respective measuring temperaturemust be known. The interfacial tension measurements required for the determination of the disperse and the polarportions of the surface tension were made with the pendant-drop method on the OCA 20. Here， too， therespective contact adhesive sample was freshly molten. By taking the molten mass into a special tubeand then pressing it out again， a drop was generated from this molten adhesive， whose contour incontact with the purely disperse liquid FC7o was then optically measured with the CCD camera andevaluated with the SCA 22 software. Here， too， the change of the curvature along the drop contourrepresenting the interface between contact adhesive and FC 7o is characteristic of the state ofequilibrium. By evaluating this drop contour according to the Laplace-Young method integrated in thesoftware， this interfacial tension between contact adhesive and FCzo can be calculated， when thedensities of the drop liquid (molten mass) and ofthe disperse liquid are known. The surface tension values for FC7o over the temperature were determined by DataPhysics by means ofown measurements made earlier. The measurements were， however， considerably complicated by the high viscosity of the contactadhesives. Some experimental skill is needed for generating a drop with a suitable contour and holdingthis drop on the tube over the entire temperature measuring rage. The manual dosing of the hot-settingadhesive drop is recommended here. Inspite of these difficulties， significant results were achieved for the examined contact adhesives in thedetermination of the total surface energies as well as of their polar and disperse portions. 5.) Summary of the liquid parameters (density，SFE -incl. FC7o) For performing the contact angle， surface tension and interfacial tension measurements on the contactadhesives，the knowledge of certain liquid parameters against the temperature is required. For the liquidsused as polar or disperse reference liquids， these data are available from DataPhysics Instruments GmbH，Filderstadt， on request.